Central projection analemmatic sundials

Construction by calculation

To design this dial by calculation, let's establish the equations giving the positions of the principal points. We will use the following system of coordinates (Fig. 3):

X axis increasing to the south, in the dial plane
Y axis increasing to the east, in the dial plane
Z axis increasing to the zenith
Origin at C, center of the equatorial circle with the radius r

In such a system, if one takes a center O of projection with coordinates xO, yO and zO then the projection of any point with coordinates x, y, and z will give a point belonging to the plane Mxy and whose coordinates x', y' and z' = 0 correspond to the double equality:

 x' - xO     y' - yO     0 - z
--------- = --------- = --------
 x - xO      y - yO      z - zO

           - zO.(x - xO)          xO.z - x.zO
so   x' = --------------- + xO = -------------
               z - zO                z - zO

          - zO.(y - yO)          yO.z - y.zO
and y' = --------------- + yO = -------------
             z - zO                z - zO

Let's now define the coordinates of the hour points of the equatorial circle and of the effective points. Suppose for a moment that we are situated at the north pole; the equator is then merged with the plane Mxy. The coordinates of the points of the circle corresponding to the hour angle h are given by the equations:

xP = - r.cos h

yP = r.sin h

with: zP = 0

and those of the effective points in accordance with the declination d of the sun by:

zP = r.tg d

with: xP = 0 et yP = 0

Now let's shift to the latitude L of the dial. The plane of the equator then turns around the y axis through the angle 90°-L. The value of the coordinate y is therefore not modified while the coordinates x and z become:

x = xP.cos (90 - L) = - r.cos h.sin L

z = xP.sin (90 - L) = - r.cos h.cos L

with: y = yP = r.sin h

Concerning the coordinates of the effective points, they become:

x = - zP.sin (90 - L) = - r.tg d.cos L

z = zP.cos (90 - L) = r.tg d.sin L

with: y = 0

We now have all the elements necessary to specify the formulas for drawing the dial. By replacing the coordinates of the hour points of the equatorial circle in the projection equations we obtain the position of the projected hour points given by:

                xo.cos L - zo.sin L
x(h) = r.cos h.---------------------
                 r.cos h.cos L + zo

          yo.cos h.cos L + zo.sin h
y(h) = r.---------------------------
             r.cos h.cos L + zo

In the same way, the position of the foot of the style is obtained by using the coordinates of the effective points:

               xo.sin L + zo.cos L
x(d) = r.tg d.---------------------
                 r.tg d.sin L - zo

                   yo.sin L
y(d) = r.tg d.-------------------
               r.tg d.sin L - zo

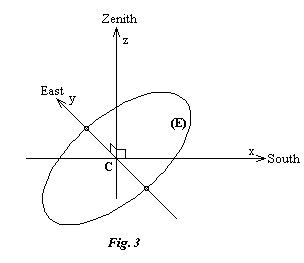
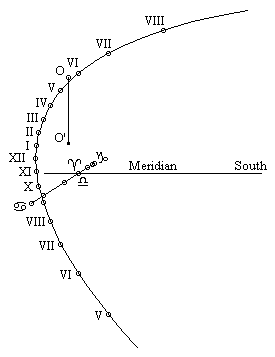
Here are some graphs of horizontal dials made for latitude 49° N and for different positions of the center O of projection. The point O' is the orthogonal projection of O on the plane of the dial.

Hyperbolic curve - The axis of rotation of the style is above the dial

Parabolic curve - The axis of rotation of the style is above the dial

Elliptical curve - the axis of rotation of the style is below the dial

Return to the main page -o- Next page

Date written: 31 August 97
Date translated: 4 February 98